DAILY DOSE of BEETHOVEN (July 8, 2020)
We now come to a real gem. Beethoven loved and admired Mozart's music. The challenge of matching it, let alone going beyond it, was daunting. Beethoven’s first great success in meeting that challenge was through his Piano Sonata No. 8, Op. 13, often called the "Pathetique." We examined several aspects of that sonata by itself on April 7-8 posts. Please feel free to revisit them.
Now let us compare it to Mozart. Beethoven's entire sonata is in a loving, and scientific dialogue with the “Fantasy in C minor, K. 475” and “Piano Sonata in C Minor K.457” by Mozart.
The “Fantasy” is often played as a prelude to the “piano sonata”, as done in the recording by Alicia de Larrocha posted on July 1st. Beethoven synopsized the entire Fantasy into a much shorter prelude.
A clear reference to the “Fantasy” is contained in the opening measures. The “Fantasy” opens with just three octaves of C, heavily stated, with middle C being the highest, deriving from Bach’s 6-part Ricercar. Beethoven's opening is also weighty, and straddles those same three octaves, but with the interstices filled out.
Mozart proceeds on to something in the second measure that we did not analyze in the last few days—a puzzling question in the higher voices: F#CEb to GBD, followed by AbCF# to GBG.
Beethoven does the same thing in his first measure, resolving from F#ACEb to GBD, but an octave lower. Just compare F#CEb-GBD, to F#ACEb-GBD. A necessarily provided audiotape helps you to hear it.
Audio: https://soundcloud.com/user-216951281/beetmozcminor1…
Is Beethoven merely quoting Mozart, or is he taking up a scientific problem?
We must examine this Lydian-Tritone question as a geometrical construction If we are to further comprehend it. The true nature of the musical system, as we have seen, had been investigated over the centuries, through a series of “12 half-steps” (chromatic scale), and the “circle of fifths”: a series of major and minor keys moving through a series of up to 12 fifths. Those investigations pushed the limits.
Now, we have something different. If you divide an octave at any but one note, you will get two different intervals. For instance, divide a C octave at G. C ascending to G is a fifth, but G ascending to C is a fourth. Try inverting those intervals. Descend by a fifth, and a fourth. Now the octave is divided at a different tone F. That is an elementary irony, and the development of that process has beautiful implications, which we addressed in our discussion of the very opening of Beethoven's Ninth Symphony on May 8th, as "Get out Your Ruler and Compass!"
The Tritone is the only interval that does not invert that way. It divides the octave exactly in half and inverts onto itself.(Ft 1). C ascending to F# is equivalent to F# ascending to C. That opens up different possibilities and ambiguities. Is F# dividing the C octave in half, or is C dividing the F# octave in half? The interval of a minor third divides the Tritone in half. C to Eb, is a minor third, as is Eb to F#(Gb). Keep ascending, and the note A functions that way. F# to a A is a minor third, as is A to C.
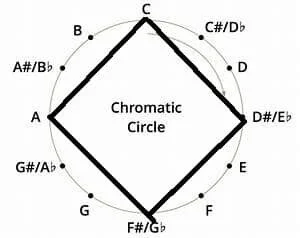
That gives you a very unstable configuration. You have two Lydian intervals C-F#, and Eb -A , and four minor thirds. What, if any, is the generating tone? This configuration can open up new pathways (Ironically, it can also be used as a cheap trick). It is also ironic, that when it is represented visually, it is very stable. Construct a circle with each of the twelve tones separated by 30 degrees, like a clock. Connect those points, C Eb F# A and C. You have inscribed a square. (See pic. 1)
Construct another such configuration by moving it up a half-tone. C Eb F# A, becomes Db E G Bb. Move up another half-step, to D F Ab B. Move up another half-step to Eb F# A C, and we have...Wait a minute! Isn't that the first one again? We have covered all twelve tones! There are only three of these things possible (Thank God!). Represent all three on a circle. (See diagram)
Sometimes a visual representation can do wonders. Notice that if we rearrange D F Ab B to B D F Ab, the outside terms, Ab to B, are our diminished 7th interval from the Musical Offering, .
Now we see three different pathways to investigate the actual nature of the musical system in which we live:
1. The investigation of half-steps, and their necessary generation of twelve tones.
C C# D D# E F F# G G# A A# B C, ascending, and C B Bb A Ab G Gb F E Eb D Db C descending. These half steps are anything but self evident.
2. The progression of the “circle of fifths”, which also generates 12 tones. They are also not self-evident, and here, the question of tempering arises once again.
C G D A E B F# Db Ab Eb Bb F C.
3. The investigation of the musical system as determined by the Lydian or Trione interval, including the double Lydian or diminished 7th. This configuration also approaches the 12 tones, but perhaps from a higher standpoint, C Eb F# A, Db F A Bb, and E G Bb Db. Have fun experimenting at the piano with it! (please refer back to the audio.)
The actual higher standpoint though, is how these harmonic systems INTERACT with one another. The best musical compositions examine this. They don't work in isolation.
We shall proceed more quickly in the next few days. An important topic has been breached!
(Ft 1). Why do we call the Tritone the "Lydian' Interval? The medieval Lydian mode went from F to F, but the 4th tone, instead of Bb, as in an F major scale, was B. Instead of a perfect 4th, F to Bb, we have an augmented 4th, F to B. That creates an instability, and a tendency for a modulation to C major to be built into the scale. When Beethoven declared the 3rd movement of his “String Quartet Op. 132” to be in the Lydian Mode, musical formalists simply refused to believe him!